ORBITADOR

Brusselator:

We consider the 1D Brusselator partial differential equation (PDE)[1]. Here we consider a state of the form $x(y,t)=(u(y,t),v(y,t))$ where $y\in \mathrm{\Omega }=[0,\ell ]$ is the spatial location. The PDE is of the form: $$\{\begin{array}{l}\frac{\partial u}{\partial t}=A+u^{2}v-(B+1)u+\sigma {\mathrm{\nabla }}^{2}u\\ \frac{\partial v}{\partial t}=Bu-u^{2}v+\sigma {\mathrm{\nabla }}^{2}v\end{array}$$ with boundary condition: $u(0,t)=u(\ell ,t)=1$, $v(0,t)=v(\ell ,t)=3$ and initial condition $x_0(y)=(u(y,0),v(y,0))$ with: $u(y,0)=1+sin(2\pi y)$, $v(y,0)=3$.
We consider: $A=1,B=3,\sigma =1/2$, $\ell =1$. We transform the PDE into a system of ODEs by spatial discretization using a grid of $N+1$ points with $N=4$ (See [2]).

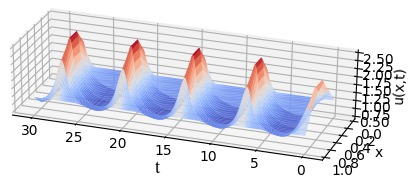
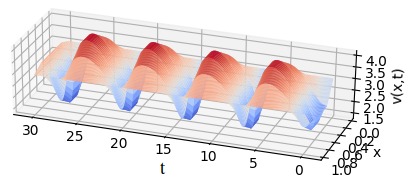
We thus consider that we have $4$ oscillators of state $x(y_i,t)\equiv (u(y_i,t),v(y_i,t))$ with initial conditions $x(y_i,0)\equiv (u(y_i,0),v(y_i,0))$ ($i=1,2,3,4$). The system of ordinary differential equations for this example is described by: $$\{\begin{array}{l}\stackrel{.}{u_1}=A+u_1^2{v}_1-(B+1)u_1+\sigma (u_0-2u_1+u_2)\\ \stackrel{.}{v_1}=B{u}_1-u_1^2{v}_1+\sigma (v_0-2v_1+v_2)\\ \stackrel{.}{u_2}=A+u_2^2{v}_2-(B+1)u_2+\sigma (u_1-2u_2+u_3)\\ \stackrel{.}{v_2}=B{u}_2-u_2^2{v}_2+\sigma (v_1-2v_2+v_3)\\ \stackrel{.}{u_3}=A+u_3^2{v}_3-(B+1)u_3+\sigma (u_2-2u_3+u_4)\\ \stackrel{.}{v_3}=B{u}_3-u_3^2{v}_3+\sigma (v_2-2v_3+v_4)\\ \stackrel{.}{u_4}=A+u_4^2{v}_4-(B+1)u_4+\sigma (u_3-2u_4+u_5)\\ \stackrel{.}{v_4}=B{u}_4-u_4^2{v}_4+\sigma (v_3-2v_4+v_5)\end{array}$$ with $u_0=u_5=1$ and $v_0=v_5=3$.

Results:

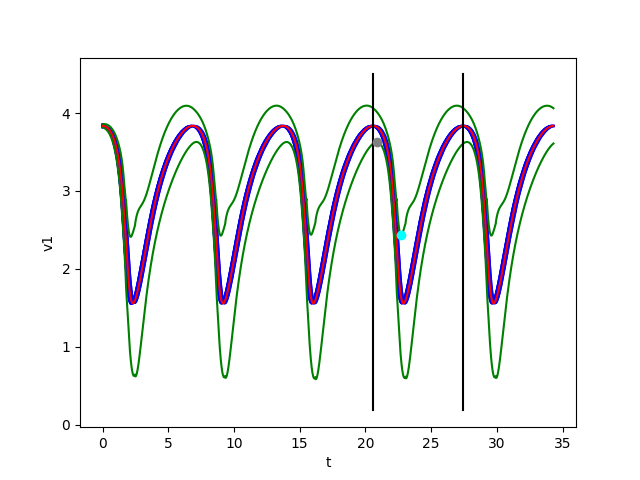
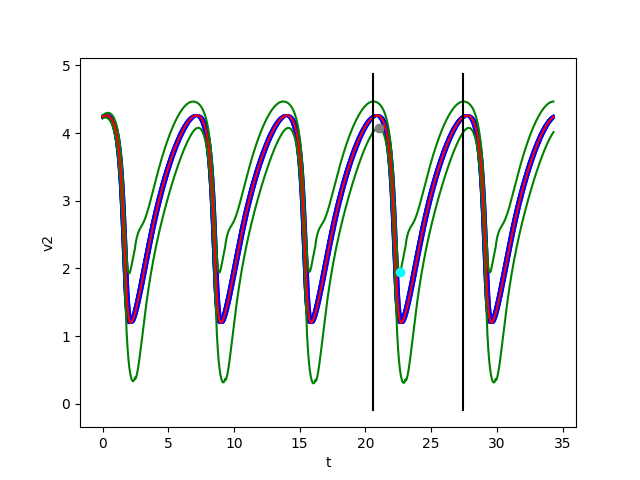
We consider a system with uncertainty $w\in \mathcal{W}=[-0.05,0.05]$, set of initial conditions $B(x_0,\epsilon )$ with $\epsilon =0.02$, the time-step used in Euler's method is $\tau =2\cdot {10}^{-4}$, and we take $T=k\tau$ with $k=34302$ as an approximate period.
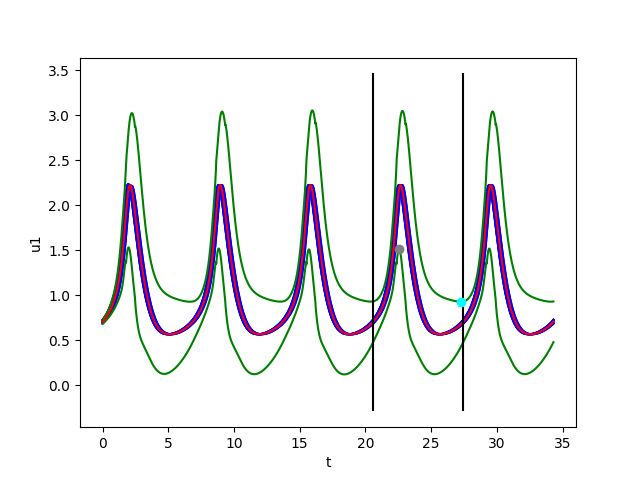
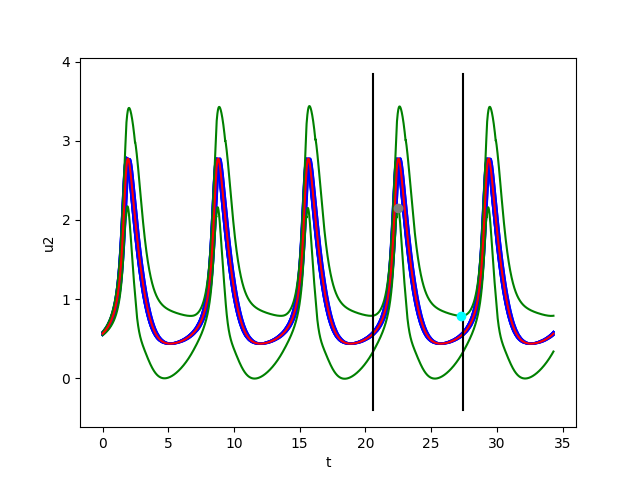
Using the figures shown below, we check that:

• $B((i_0+1)T)\subset B(i_{0}T)$ for $i_0=3$
• also for $u_1$, the minimum $m_{+}^1=0.92679$ (represented by a small cyan ball) of the upper green curve ${\tilde{u}}_1(t)+{\delta }_{\mathcal{W}}(t)$ is less than the maximum $M_{-}^1=1.51263$ (represented by a small gray ball) of the lower green curve ${\tilde{u}}_1(t)-{\delta }_{\mathcal{W}}(t)$
• and ${\mathrm{\Sigma }}_{i=1}^k{\lambda }_i\approx$ $-27147.716<0$.

Then, we can conclude that the system converges towards an attractive LC contained in $[B(3T),B(4T)]$.

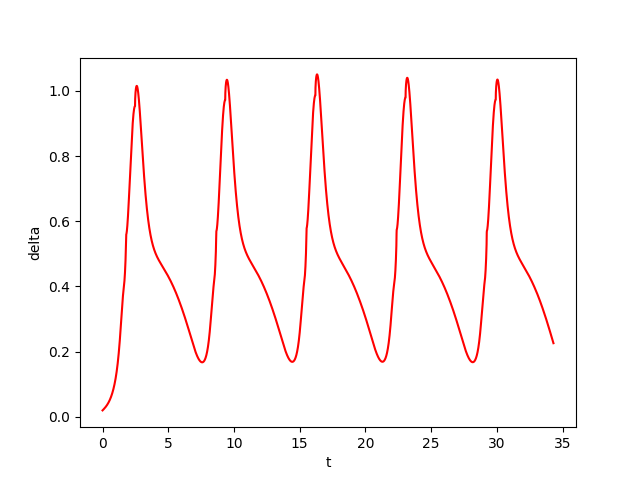
The figures below show respectively the simulation of $u_1(t),u_2(t),v_1(t),v_2(t)$ and ${\delta }_{\mathcal{W}}(t)$ with perturbation (w=0.05) over 5 periods (5T=34,3) for dt=0.0002. The red curves represent the Euler approximation, the green curves correspond to the borders of tube $B_w$. The black vertical lines delimit the portion of the tube between $t=i_0\ast T_0$ and $t=(i0+1)T_0$. The cyan point represents the minimum $m_{+}^1$ of the upper green curve and the gray point shows the maximum $M_{-}^1$ of the lower green curve.

To get more results and details, Click Here

References:

[1] CHARTIER, Philippe and PHILIPPE, Bernard. A parallel shooting technique for solving dissipative ODE's. Computing, 1993, vol. 51, no 3-4, p. 209-236..
[2] JERRAY, Jawher, FRIBOURG, Laurent, and ANDRÉ, Étienne. Guaranteed phase synchronization of hybrid oscillators using symbolic Euler's method (verification challenge). EPiC Series in Computing, 2020, vol. 74, p. 197-208.