ORBITADOR

Lorenz:

The following system of differential equations was introduced by Lorenz (see [1]): $$\{\begin{array}{l}\stackrel{.}{x_1}=-\sigma x_1+\sigma x_2\\ \stackrel{.}{x_2}=\rho x_1-x_2-x_1{x}_3\\ \stackrel{.}{x_3}=-\beta x_3+x_1{x}_2\end{array}$$

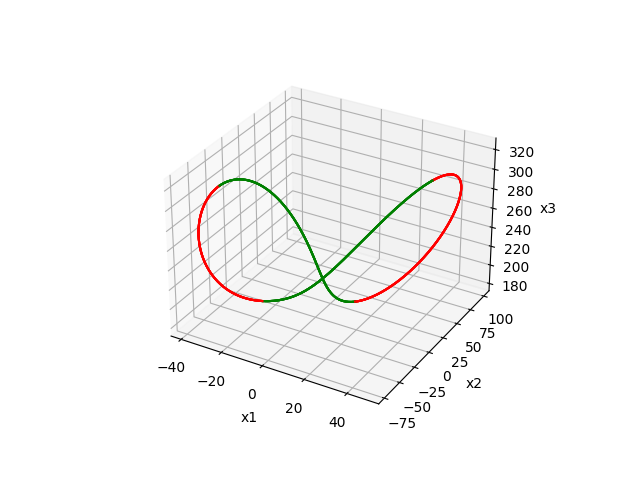
As a crude model of atmospheric dynamics, these equations led Lorenz to the discovery of sensitive dependence of initial conditions—an essential factor of unpredictability in many systems.
We consider: the classical parameter values $\sigma =10,\beta =8/3$, $\rho =250$ and initial condition $x(0)=(16.21325444114593,-55.78140243373939,249)$ (See [2])

Results:

We consider a system without uncertainty $w=0$, set of initial conditions $B(x_0,\epsilon )$ with $\epsilon =350$, the time-step used in Euler's method is $\tau ={10}^{-5}$, and we take $T=k\tau$ with $k=46000$ as an approximate period.
Using the figures shown below, we check that:

• $B((i_0+1)T)\subset B(i_{0}T)$ for $i_0=2$
• and ${\mathrm{\Sigma }}_{i=1}^k{\lambda }_i\approx$ $-6.304<0$.

Then, we can conclude that the system converges towards an attractive LC contained in $[B(2T),B(3T)]$.

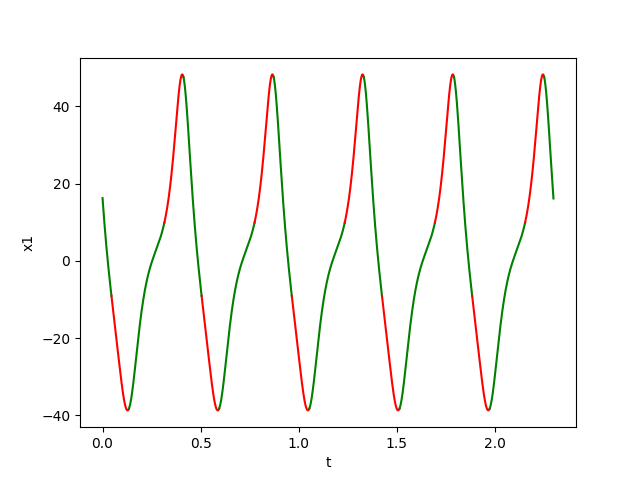
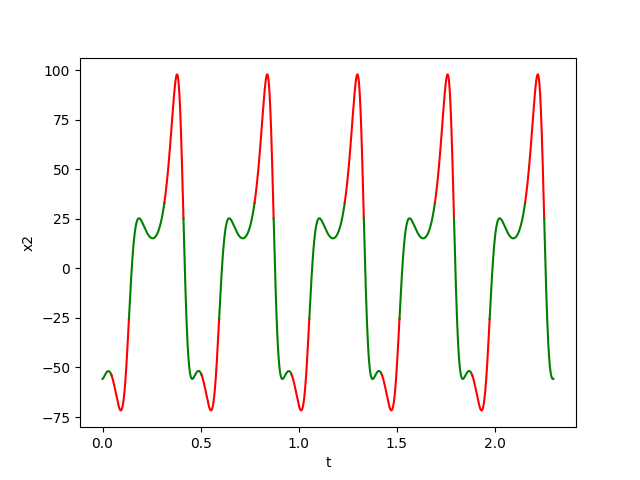
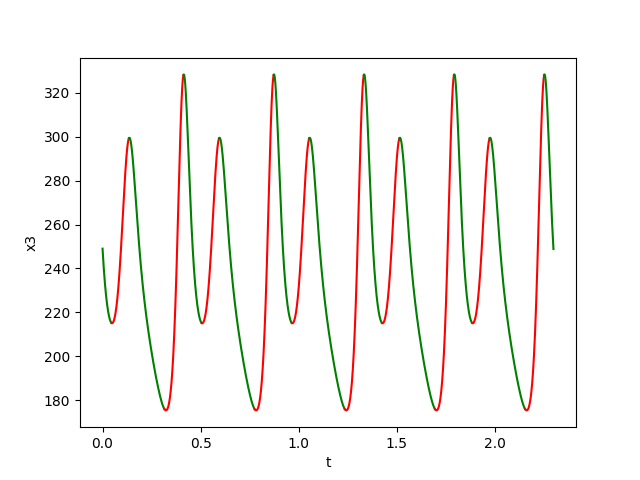
The figures below show respectively the simulation of $x_1(t),x_2(t),x_3(t)$ and ${\delta }_{\mathcal{W}}(t)$ without perturbation (w=0) over 5 periods (5T=2.3) for dt=0.00001.

References:

[1] LORENZ, Edward N. Deterministic nonperiodic flow. Journal of atmospheric sciences, 1963, vol. 20, no 2, p. 130-141.
[2] TUCKER, Warwick. Computing accurate Poincaré maps. Physica D: Nonlinear Phenomena, 2002, vol. 171, no 3, p. 127-137.